
About
Currently (2022 - present), I’m a Research Engineer at Global Engineering and Materials, Inc. at Princeton, New Jersey.
I am a computational fluid dynamicist with rich industrial and academic experience in diverse fluid mechanics and heat transfer problems. Throughout my research and development career, I have utilized a variety of CFD tools (commercial CFD codes such as StarCCM+ and in-house CFD codes such as Lattice Boltzmann code, JHU’s LES code, and MIT’s general circulation code) to explore a wide range of complex fluid dynamics problems.
My current research activities are industry-focused aiming to develop high-fidelity process-simulation tools to capture multi-scale, multi-physics processes occurring in the autoclave and out-of-autoclave environments that are widely used in aircraft structure manufacturing industries, and phase transformation processes occurring in the quenching of aerospace aluminimum forging parts. My responsibilities include developing CFD models to capture complex fluid physics, analyzing results, collaborating with experimentalists to design test cases, identifying and rectifying design issues and making design recommendations, validating against benchmark problems as well as test data, writing technical reports and papers, and conducting team meetings within the company and with external collaborators. The SBIR-sponsored research projects I am involved in are in collaboration with various academic and industry partners sponsored by the program.
My past academic experiences cover a broad spectrum of research fields related to mechanical engineering and physical oceanography. My work explored the physics of boundary layer turbulent processes in the ice-free/ice-aware upper ocean and atmospheric surface layer and in developing parameterization schemes for climate models to improve the predictability of Earth’s environment. Earth's geophysical fluid dynamical processes have length scales and time scales that span over several orders of magnitude, and all of these processes in all scales can interact with each other, making this a challenging system to solve. At the University of Washington-Seattle, my work was focused on understanding the interactions between sea ice and submesoscale ocean variability. I used ocean-ice models to design idealized high-resolution numerical experiments to explore the underlying physics and devise parameterizations relevant to improving the predictive capabilities of the models of the rapidly changing polar regions. At UT Dallas, my research focussed on understanding the kinematic characteristics of Langmuir turbulence in coastal zones and developing theoretical models to assess the coherence and strength of coastal Langmuir Circulations. My research efforts at Lille 1 University, France contributed to the development of three-dimensional Lattice Boltzmann code to study convective, turbulent flows.
Research
1. Submesoscale-Induced Basal Melting of Ice Shelf Cavities in Polar Regions
Several ice shelves in the Arctic’s and Antarctica’s coastlines have dramatically collapsed in very recent times (e.g. Brunt Ice Shelf - February 26, 2021; Milne Ice Shelf -August 4, 2020; Pine Island Glacier - February 2020, etc.). Such events can result in global sea level rise (around 0.24 inches rise was recorded from just 2018 to 2019) and pose an increased threat to the world’s coastal population. For an accurate assessment of ocean-forced glacier mass loss, global ocean models need to incorporate all key processes that bring heat toward the ice shelf base and enhance melting. Coarse-resolution climate projection models may be under-representing one of the key influences from the ocean eddies of a few km in size. In this study, we provide an argument that the effect of those eddies is significant and needs to be accounted for more accurate predictions of ice shelf basal melting and future projections of global sea level rise.
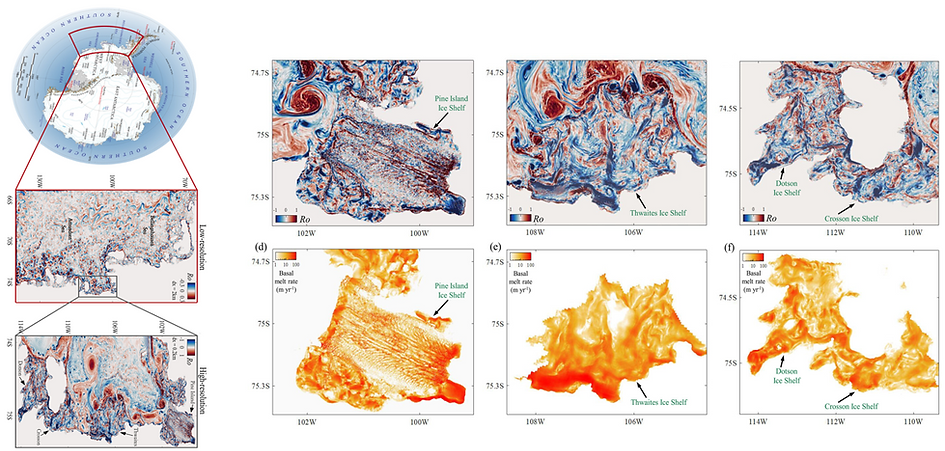
Ice shelf cavities in the Antarctica region highlighting highest basal melting near the grounding line
2. Physical Processes In The Upper Ocean
Earth's climate and ecosystem are mainly governed by three components: ocean, atmosphere, and sea ice, and the coupled interactions between them. Each of these components is comprised of a large number of physical, chemical, and biological processes with length scales and time scales varying over several orders of magnitude, and all these processes in all scales interact with each other. Thus, the Earth system is a non-linear, complex, gigantic problem to solve. One of the viable research techniques to address such a problem is the global climate model (GCM), where the Earth system processes are represented with mathematical equations and computationally solved using supercomputers. The equations are solved in a 3D grid of cells that cover the Earth's surface. Hence, it is easy to understand that the processes larger than the model grid size are fully resolved, and the subgrid-scale processes need to be parameterized.
Ocean surface mixed layer acts as a gateway between the atmosphere, sea ice, and ocean interior, mediating the exchange of heat, mass, momentum, and other tracers, and thus plays a crucial role in global climate. My research is focused on two of the most important surface mixed layer processes: (1) Ocean boundary layer phenomenon called Langmuir Circulations (2) Submesoscale mixed layer restratification. Since both of these processes are typically smaller than the GCM model resolution in terms of length scales, thus I am interested in exploring their underlying physics and developing/improving parameterization schemes for climate models.
a. Submesoscale Mixed Layer Restratification Under Sea Ice
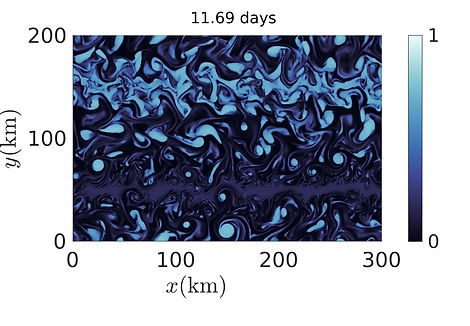
c = 0.2
Idealized simulation of frontal spindown in marginal ice zones
sea ice concentration
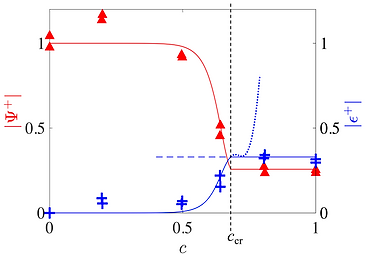
Overturning streamfunction vs. sea ice concentration
The ocean surface is filled with fronts that can become unstable to ageostrophic baroclinic instabilities and lead to the formation of submesoscale (SM) eddies in the ocean mixed layer (ML). These instabilities convert the lateral density gradients into vertical gradients and overturn the density fronts releasing potential energy. It is known that the vertical fluxes due to SM ML eddies play a significant role in the ML buoyancy budget. Also, the restratification process associated with the overturning of the fronts strongly influences variations in the ML depth. Hence, understanding the ML restratification process is of first-order importance in improving the accuracy of global climate models.
Fox-Kemper and Ferrari (JPO, 2008) showed that the MLE-induced restratification process could be cast in terms of an eddy-driven overturning streamfuntion such that eddy fluxes of density and other tracers can be approximated. Given its simple formulation and its accuracy in predicting changes to the buoyancy, it has been incorporated in many, if not all, climate projection models.
Parameterization of Fox-Kemper and Ferrari (JPO,2008)
:
where, are ML depth, lateral buoyancy gradients and the Coriolis parameter
is the vertical structure of overturning streamfunction
= 0.06-0.08
In our study, we found that the presence of sea ice cover induces a strong coupling between sea ice and surface ocean and hence strongly affects the ML restratification process. However, the conventional parameterization does not take into account the presence of sea ice in any way while being used in ice-covered polar oceans. Thus, we developed an improvement to the existing ML overturning parameterization, making it explicitly dependent on sea ice concentration,
Our parameterization scheme under sea ice
:
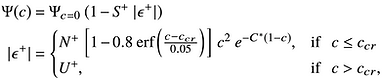
where,
The updated parameterization scheme predicts the reduction of the overturning streamfunction up to a factor of 4 for fully ice-covered regions with the sharp transition of submesoscale eddy characteristics occurring at critical sea ice concentration. The inclusion of such parameterization in global climate models can improve the representation of ocean-ice interactions and consequently lead to more accurate sea ice predictions.
b. Coastal-zone Langmuir Turbulence

Effect of oblique wind (blue arrow), wave (red) and mean current (black) forcing on LC orientation
The ocean boundary layer (OBL) occupies the upper ≈100 m of Earth’s oceans and is forced by atmosphere-ocean and sea ice-ocean momentum fluxes, wave dynamics, and buoyancy. Foremost among OBL flow states is Langmuir turbulence – formed in response to simultaneously present wind and wave loading – resulting in the formation of streamwise-elongated, counterrotating Langmuir cells (LC). LC is a key contributor to vertical mixing and transport of materials in the upper mixed layer of the ocean and significantly contributes to physical and geochemical processes relevant to oil spill turbulent dispersion, ecology in the upper ocean, and parameterization of climate models. LC in coastal regions is an even more complex phenomenon further influenced by bottom-boundary layer shearing. Coastal-zone Langmuir turbulence is of pivotal importance to sediment erosion and resuspension, turbidity, dispersion of anthropogenic quantities, coastal biodiversity, etc. however, only a minority of preceding studies have addressed its characteristics.
Our study on the characteristics of LC in coastal regions have led to some interesting findings that have been summarized below:
(1) We presented evidence that intense downwelling and upwelling within the coastal LC induce phase-locked modulation of bathymetric stress.
(2) We explored the distinctive structural attributes of coastal LC compared to open-ocean LC and the ''cause and effect'' dynamics of wind and wave forcing (wind stress, wavenumber of the surface waves, and Stokes drift surface velocity) with their spatial length scales.
(3) We showed that oblique forcing between mean current associated with tidal currents, surface wave field, and atmospheric wind current in coastal zones influence the orientation of resultant coastal LC and provided theoretical justifications for the numerical results. Also, a model to predict the orientation was proposed.
Prognostic model to predict resultant LC orientation angle:
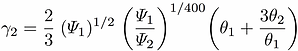
where,
= ratio of wind shear velocity to the bottom-wall friction velocity
= ratio of surface Stokes drift velocity to the bottom-wall friction velocity
= angle between wind stress and mean current forcing direction
= angle between Stokes drift vector and wind forcing direction
3. Lattice Boltzmann Method for Turbulent Flows
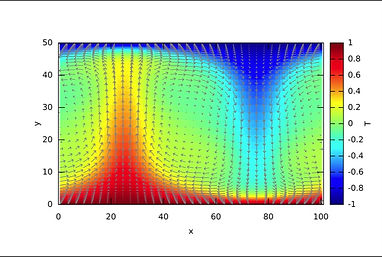
Simulation of Rayleigh-Benard convection using FV LB method
Lattice Boltzmann Method (LBM) has emerged as a viable alternative to Navier-Stokes Direct Numerical Simulations (NS DNS) in fluid dynamics research. It is based on the mesoscopic level of the description that goes beyond the Navier-Stokes continuum matter description of fluids and can handle complex effects, such as the interaction between different fluid components, phase-change processes, and non-Newtonian rheology, with much ease compared to other macroscopic methods. Also, the LB method has very appealing features from a computational point of view. It is simple to implement, free
of numerical diffusion and stability issues, and suitable for parallelization due to its local-in-space character. However, one major shortcoming comes from the limitation to spatially cubic grids, which becomes particularly critical in the simulation of realistic turbulent flows where near-to-wall grid refinement is needed. To address the limitation of the standard streaming LB algorithm, we developed a novel finite-volume (FV) discretization method for the LB equation, which besides a high level of accuracy, also displays a contained computational cost.
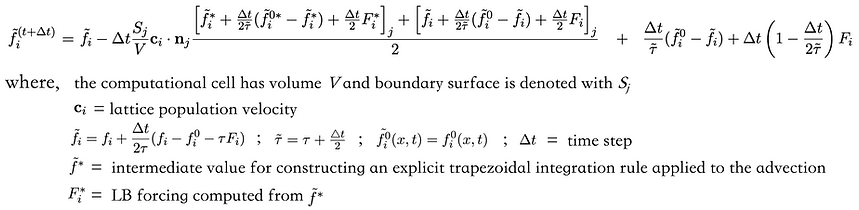
The developed FV LB method was methodically and carefully compared with the standard streaming LB method using an in-house LB code ''ch4-project'' where both the above-mentioned LB algorithms coexist simultaneously. The ''ch4-project'' LB code is written in ''C'' language and parallelized using MPI and has been validated upon a variety of laminar and turbulent flows, including the turbulent Rayleigh-Benard convection system. It is a general-purpose LB code that can address a variety of fluid dynamics problems. Simulating heavy/light gas dispersion in the atmosphere is one among others.

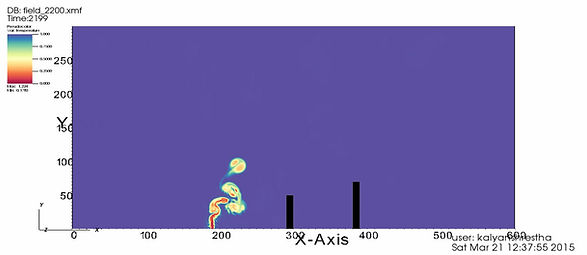
4. Jet Injected in a Supersonic Crossflow

Winner of the "CFD movie of the year 2010-2011, Cranfield U"
Flapless maneuvering of missiles and rockets, fuel injection into a scramjet combustion chamber, and noise reduction in wheel bays - what do these have in common? They all rely on sonic microjets injecting high-speed gas into a free stream.
Our study investigated the ability of modern, sophisticated RANS (Reynolds Averaged Navier Stokes) methods to accurately represent jet injection. This video won the ''video of the year in 2010-2011'' and highlights the flow features captured in the CFD simulations. We also examined mixing rates that are important for combustion and the accuracy of predicted pressure distributions - of prime importance in actuation applications.
(12) Xu Y, Zhao Z, Shrestha K, Seneviratne W, Liyanage S, Palliyaguru U, Karuppiah A, Lua J, Phan N, Yan J (2024) A coupled data-physics computational framework for temperature, residual stress, and distortion modeling in autoclave process of composite materials, Composites Part A: Applied Science and Manufacturing 183, 108218.
(11) Shrestha K, Manucharyan G. E., Nakayama Y. (2024) Submesoscale Variability and Basal Melting in Ice Shelf Cavities of the Amundsen Sea, Geophysical Research Letters 51, e2023GL107029.
(10) Shrestha K, Karuppiah A, Liu N, Lua J (2023) Towards a Digital Twin for High-Quality Curing of Multiple Composite Parts in Autoclave, Proceedings of the American Society for Composites - Thirty - Eighth Annual Technical Conference, DOI: 10.12783/asc38/36679.
(9) Lua J, Karuppiah A, Li X, Shrestha K, Yan J, Zhao Z, Zhang D (2023) A Multiphysics Coupling for Evaluation of Effects of Local Boundary Conditions on Autoclave-Cured Composite, AIAA SCITECH 2023 Forum, 0524.
(8) Shrestha K, Enos R, Li X, Wei Q, Elenchezhian, Zhang D, Lua J (2023) Integrated flow and stress modeling for infusion and curing process in Liquid Composite Molding, Proceedings of the American Society for Composites - Thirty - Seventh Annual Technical Conference. DOI: 10.12783/asc37/36399.
(7) Shrestha K, Manucharyan G. E. (2022) Parameterization of submesoscale mixed layer restratification under sea ice, Journal of Physical Oceanography 52(3), pp. 419-435.
(6) Shrestha K, Anderson W (2020) Coastal Langmuir circulations induce phase-locked modulation of bathymetric stress, Environmental Fluid Mechanics 20, pp.873–884.
(5) Shrestha K, Anderson W, Tejada-Martinez A, Kuehl J (2019) Orientation of coastal-zone Langmuir cells forced by wind, wave, and mean current at variable obliquity, Journal of Fluid Mechanics 879, pp.716–743.
(4) Anderson W, Yang J, Shrestha K, Awasthi A (2018) Turbulent secondary flows in wall turbulence: vortex forcing, scaling arguments, and similarity solution, Environmental Fluid Mechanics 18(6), pp.1351–1378.
(3) Shrestha K, Anderson W, Kuehl J (2018) Langmuir turbulence in coastal zones: structure and length scales, Journal of Physical Oceanography 48(5), pp.1089–1115.
(2) Ibanez R, Kuehl J, Shrestha K, Anderson W (2018) Brief Communication: A nonlinear self-similar solution to barotropic flow over varying topography, Nonlinear Processes in Geophysics 25(1), pp.201–205.
(1) Shrestha K, Mompean G, Calzavarini E (2016) Finite-volume versus streaming-based Lattice Boltzmann algorithm for fluid-dynamics simulations: A one-to-one accuracy and performance study, Physical Review E 93(2), 023306.
Theses
Shrestha K (2015) Simulation of wall-bounded turbulent convective flows by Finite Volume Lattice Boltzmann Method, PhD Thesis, Lille 1 University of Sciences and Technologies, Lille, France.
Advisors: Enrico Calzavarini, Gilmar Mompean
Shrestha K (2011) Investigation on the accuracy of RANS models for under-expanded jet injected into a supersonic cross-flow, MSc Thesis, Cranfield Univesity, UK.
Advisors: Ben Thornber, Zeeshan Rana


